Nonlinear Optimal and Multi-Loop Flatness-Based Control for the 6-DOF Autonomous Bicopter

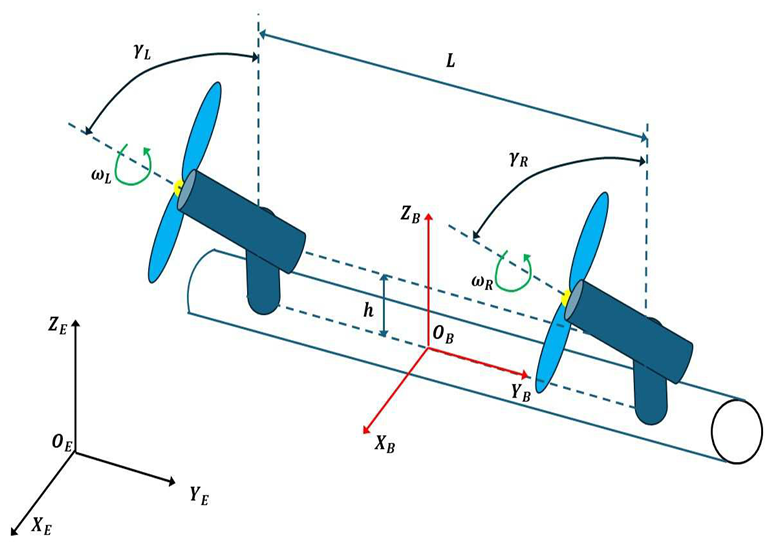
Bicopter UAVs can find use in several civilian and defence applications. In the present article a solution of the nonlinear optimal control problem of 6-DOF bicopters is first attempted using a novel nonlinear optimal control method. This method is characterized by computational simplicity, clear implementation stages and proven global stability properties. At a first stage, approximate linearization is performed on the dynamic model of the 6-DOF bicopter with the use of first-order Taylor series expansion and through the computation of the system’s Jacobian matrices. This linearization process is carried out at each sampling instance, around a temporary operating point. At a second stage, an H-infinity stabilizing controller is designed for the approximately linearized model of the 6-DOF bicopter. To find the feedback gains of the controller an algebraic Riccati equation is repetitively solved, at each time-step of the control method. Lyapunov stability analysis is used to prove the global stability properties of the control scheme. Next, the article examines a multi-loop flatness-based control method for the dynamic model of the 6-DOF bicopter. The drone’s dynamics is written in the form of two chained subsystems which are shown to be differentially flat. The state vector of the second subsystem becomes virtual control input to the first subsystem, while the control inputs of the first subsystem become setpoints for the second subsystem. Local controllers for the individual subsystems invert their dynamics. The global stability properties of the multi-loop flatness-based control scheme are also proven though Lyapunov analysis.